Mathematicians say that a torus (donut) can be created by starting with a rectangle and connecting opposite sides of a rectangle. As part of a summer project, a team of Bryn Mawr students figured how to do this construction using math formulas. This torus video shows how.
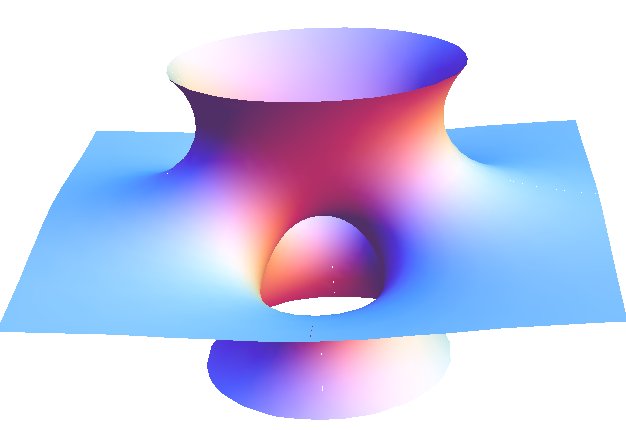
In the 1980s, the Brazilian mathematician Manifrido Costo discovered a surprisingly shaped surface (now called the Costa surface) with the unusual property that it is a “minimal” surface which can be extended forever without intersecting itself. This surface can be thought of as a torus with three holes even though it may not look much like a torus at first glance. The Costa surface was a key part of the math exhibit Beyond Numbers staged at the Maryland Science Center. The exhibit designers were looking for a video which would show the relationship between the torus and the Costa surface. Bryn Mawr math major Mihaela Teoderescu was working on just such a project for her senior math thesis which resulted in this Costa video which become part of the Beyond Numbers exhibit.