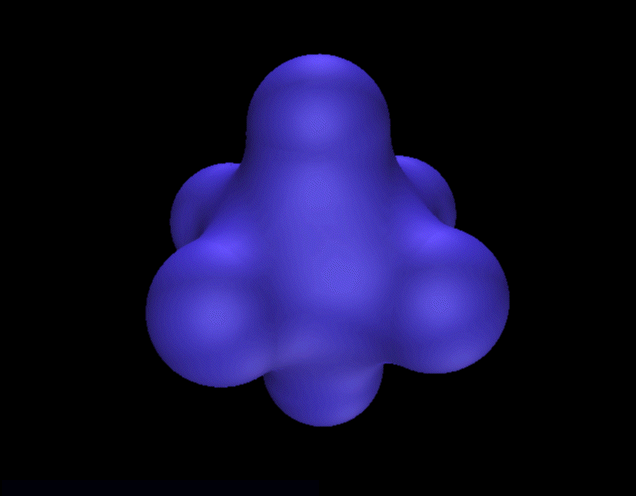
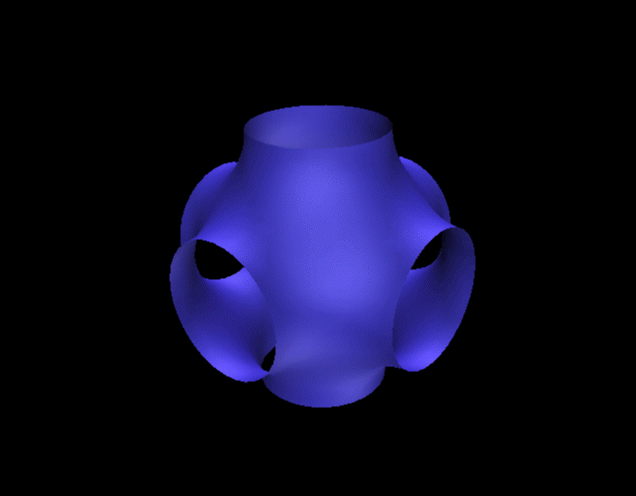
Sphere with Chaotic Geodesic Flow
My area of research is chaotic properties of dynamical systems well as understanding the transition between regular and chaotic motions. Systems that I am particularly interested include geodesic flow on surfaces and billiards. My colleague Keith Burns and I created the first examples of (unusual shaped) spheres and other surfaces that can actually exist in regular three-dimensional space and whose geodesic motion is chaotic (Embedded surfaces with ergodic geodesic flow, Inter. J. of Bifurcation and Chaos Vol. 7, No. 7 (1997), 1509-1527.) Below are more such surfaces. These examples are “unstable”: under a small perturbation to the surface, the chaotic motion can switch to having regions of stable motion (Destroying ergodicity in geodesic flows on surfaces, Nonlinearity 19 (2006) 149-169).
More recently, in collaboration with Charles Pugh (Anosov geodesic flows for embedded surfaces, Astérisque 287 (2003), 61-69, in Geometric methods in Dynamics II) and with Daniel Visscher (A new proof of the existence of embedded surfaces with Anosov geodesic flow, Regular and Chaotic Dynamics, 23 (6):685-694), we have made examples of surfaces in three-dimensional space with “stable” chaotic behavior: the chaotic behavior remains even if the shape of the surface is changed slightly. Information on other of my articles can be found here.
The P-surface a minimal surface with the symmetry of a cube.
By attaching "focusing caps" to each of the ends, we create a sphere. This is the first known example of a sphere in three dimensional space whose geodesic flow is chaotic (ergodic).
Connecting four copies of the P-surface produces this shape.
Attaching caps to the ends produces a torus whose geodesic flow is also chaotic. Taking more and more copies of the P-surface allows one to make surfaces of arbitrary genus.
Images created by Bryn Mawr math major Louisa Winer using Mathematica, Surface Evolver and Geomview. Thanks to Rob Kusner, Ken Brakke, Bogdan Butoi, Michelle Francl and Lisa Chirlian for technical assistance.